Solutions For All Chapters Maths Class 7
Ex 4.3 Class 7 Maths Question 1.
Set up equations and solve them to find the unknown numbers in the following cases:
(a) Add 4 to eight times a number; you get 60.
(b) One-fifth of a number minus 4 gives 3.
(c) If I take three-fourths of a number and add 3 to it, I get 21.
(d) When I subtracted 11 from twice a number, the result was 15.
(e) Munna subtracts thrice the number of notebooks he has from 50, he finds the result to be 8.
(f) Ibenhal thinks of a number. If she adds 19 to it and divides the sum by 5, she will get 8.
(g) Anwar thinks of a number. If he takes away 7 from 5/2 of the numbers, the result is 23.
Solution:
(a) Let the required number be x.
Step I: 8x + 4
Step II: 8x + 4 = 60 is the required equation
Solving the equation, we have
8x + 4 = 60
⇒ 8x = 60 – 4 (Transposing 4 to RHS)
⇒ 8x = 56
⇒ 8 x/8 = 56/8 (Dividing both sides by 8)
⇒ x = 7
Thus, x – 7 is the required unknown number.
(b) Let the required number be x.
Step I: 1/5 x – 4
Step II: 1/5 x – 4 = 3 is the required equation. 5
Solving the equation, we get
1/5 x – 4 = 3
⇒ 1/5 x = 4 + 3 (Transposing 4 to RHS)
⇒ 1/5 x = 7
⇒ 1/ 5 x × 5 = 7 × 5 (Multiplying both sides by 5)
⇒ x = 35 is the required unknown number,
(c) Let the required number be x.
Step I: 3 /4 x + 3
Step II: 3 /4 x + 3 = 21 is the required equation.
Solving the equation, we have
⇒ x = 24 is the required unknown number.
(d) Let the required unknown number be x.
Step I: 2x – 11
Step II: 2x -11 = 15 is the required equations.
Solving the equation, we have
2x – 11= 15
⇒ 2x = 15 + 13 (Transposing 11 to RHS)
⇒ 2x = 28
⇒ 2 x/2 = 28/2 (Dividing both sides by 2)
⇒ x = 14 is the required unknown number,
(e) Let the required number be x.
Step I: 50 – 3x
Step II: 50 – 3x = 8 is the required equations.
Solving the equation, we have
50 – 3x = 8
⇒ -3x = 8 – 50 (Transposing 50 to RHS)
⇒ -3x = -42
⇒ -3 x/-3 = -42/-3 (Dividing both sides by -3)
⇒ x = 14 is the required unknown number.
(f) Let the required number be x.
Step I: x + 19
Step II: x + 19/5
Step III: x + 19/5 = 8 is the required equation.
Solving the equation, we have
x + 19/5 = 8
⇒ x + 19/5 × 5 = 8 × 5(Multiplying both sides by 5)
⇒ x + 19 = 40
⇒ x = 40 – 19 (Transposing 19 to RHS)
∴ x = 21 is the required unknown number.
(g) Let the required number be x.
Step I: 5/2x – 7
Step II: 5/5 – 7 = 23 is the required equation.
Solving the equation, we have
⇒ x = 12 is the required unknown number.
Ex 4.3 Class 7 Maths Question 2.
Solve the following:
(a) The teacher tells the class that the highest marks obtained by a student in her class is twice the lowest marks plus 7. The highest score is 87. What is the lowest score?
(b) In an isosceles triangle, the base angle are equal. The vertex angle is 40°. What are the base angles of the triangle? (Remember, the sum of three angles of a triangle is 180°?)
(c) Sachin scored twice as many runs as Rahul. Together, their runs fell two short of a double century. How many runs did each one score?
Solution:
(a) Let the lowest score be x.
Step I: Highest marks obtained = 2x + 7
Step II: 2x + 7 = 87 is the required equation. Solving the equation, we have
2x + 7 = 87
⇒ 2x = 87 – 7 (Transposing 7 to RHS)
⇒ 2x = 80
⇒ 2 x/2 = 80/2 (Dividing both sides by 2)
⇒ x = 40 is the required lowest marks.
(b) Let each base angle be x degrees.
Step I: Sum of all angles of the triangle (x + x + 40) degrees.
Step II: x + x + 40 = 180°
⇒ 2x + 40° = 180°
Solving the equation, we have
2x + 40° = 180°
2x = 180° – 40° (Transposing 40° to RHS)
2x = 140°
⇒ 2 x/2 =140°/2 (Dividing both sides by 2)
⇒ x = 70°
Thus the required each base angle = 70°
(c) Let the runs scored by Rahul = x
Runs scored by Sachin = 2x
Step I: x + 2x = 3x
Step II: 3x + 2 = 200
Solving the equation, we have
3x + 2 = 200
⇒ 3x = 200 – 2 (Transposing 2 to RHS)
⇒ 3x = 198
⇒ 3 x/ 3 = 198/3 (Dividing both sides by 3)
⇒ x = 66
Thus, the runs scored by Rahul is 66 and the runs scored by Sachin = 2 × 66 = 132
Ex 4.3 Class 7 Maths Question 3.
Solve the following:
(i) Irfan says that he has 7 marbles more than five times the marbles Parmit has. Irfan has 37 marbles. How many marbles does Parmit have?
(ii) Laxmi’s father is 49 years old. He is 4 years older than three times Laxmi’s age. What is Laxmi’s age?
(iii) People of Sundargram planted trees in a village garden. Some of the trees were fruit trees. The number of non-fruit trees were two more than three times the number of fruit trees. What was the number of fruit trees planted if the number of non-fruit trees planted was 77?
Solution:
(i) Let the number of marbles with Parmit be
Step I: Number of marbles that Irfan has = 5x + 7
Step II: 5x + 7 = 37 Solving the equation, we have 5x + 7 = 37
⇒ 5x = 37 – 7 (Transposing 7 to RHS)
⇒ 5x = 30
⇒5 x/5 = 30/5 (Dividing both sides by 5)
⇒ x = 6
Thus, the required number of marbles = 6.
(ii) Let Laxmi’s age be x years.
Step I: Father’s age = 3x + 4
Step II: 3x + 4 = 49
Solving the equation, we get
3x + 4-= 49
⇒ 3x = 49 – 4 (Transposing to RHS)
⇒ 3x = 45
⇒ 3 x/3 = 45/3 (Dividing both sides by 3)
⇒ x = 15
Thus, the age of Laxmi = 15 years
(iii) Let the number of planted fruit tree be x.
Step I: Number of non-fruit trees = 3x + 2
Step II: 3x + 2 = 77
Solving the equation, we have
3x + 2 = 77
⇒ 3x = 77 – 2 (Transposing 2 to RHS)
⇒ 3x = 75
⇒ 3 x/ 3 = 75/3 (Dividing both sides by 3)
⇒ x = 25
Thus, the required number of fruit tree planted = 25
Ex 4.3 Class 7 Maths Question 4.
Solve the following riddle:
I am a number,
Tell my identity!
Take me seven times over
And add a fifty!
To reach a triple century
You still need forty!
Solution:
Suppose my identity number is x.
Step I: 7 + 50
Step II: 7x + 50 + 40 = 300
or 7x + 90 = 300
Solving the equation, we have
7x + 90 = 300
⇒ 7x = 300 – 90 (Transforming 90 to RHS)
⇒ 7x = 210
⇒ 7x/7 = 210/7 (Dividing both sides by 7)
⇒ x = 30
Thus, my identity is 30.

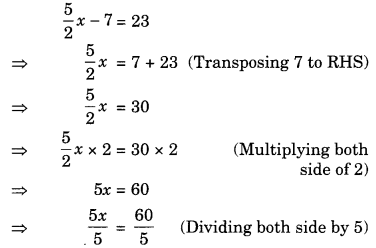

Leave a Reply