Large Numbers Around Us
Page No. 2
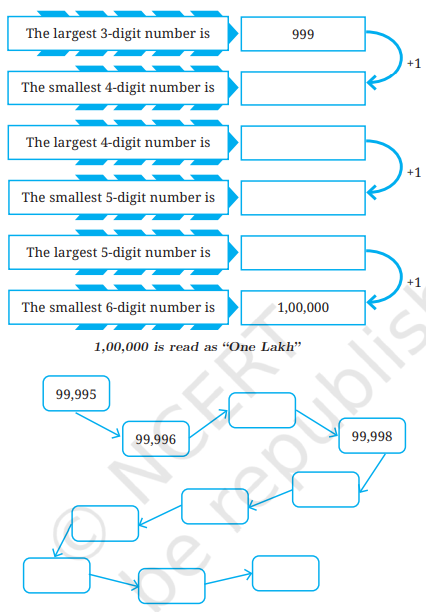
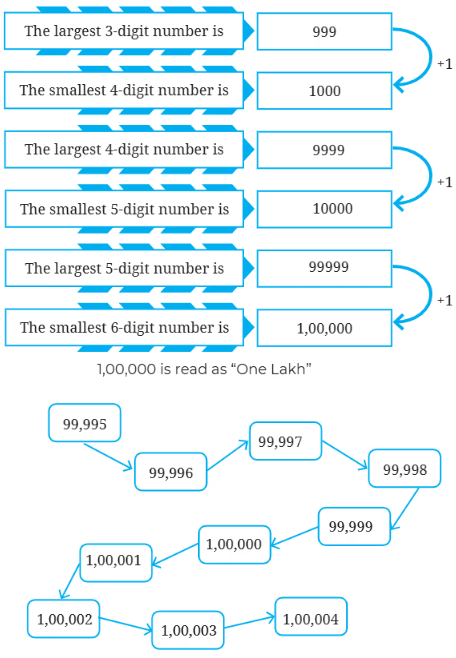
Question: But how much is one lakh? Observe the pattern and fill in the boxes given below.
Answer:
Question: Roxie and Estu found that if they ate one variety of rice a day, they would come nowhere close to a lakh in a lifetime! Roxie suggests, “What if we ate 2 varieties of rice every day? Would we then be able to eat 1 lakh varieties of rice in 100 years?”
Solution: To find out, calculate the number of rice varieties eaten in 100 years.
- Number of days in a year = 365 (ignoring leap years).
- Number of days in 100 years = 365 × 100 = 36,500 days.
- Varieties eaten per day = 2.
- Total varieties in 100 years = 36,500 × 2 = 73,000.
- One lakh = 100,000.
Since 73,000 is less than 100,000, they cannot eat 1 lakh varieties in 100 years.
Question: Choose a number for y. How close to one lakh is the number of days in y years, for the y of your choice?
Solution: Let’s choose y = 274 years.
- Number of days in 1 year = 365.
- Number of days in 274 years = 365 × 274 = 100,010.
- One lakh = 100,000.
- Difference = 100,010 − 100,000 = 10.
The number of days (100,010) is very close to one lakh, just 10 days more.
Figure it Out
Page No. 3
1. According to the 2011 Census, the population of the town of Chintamani was about 75,000. How much less than one lakh is 75,000?
Solution:
- Population of Chintamani in 2011 = 75,000.
- One lakh = 1,00,000.
- We need to find how much less 75,000 is than 1,00,000.
Calculate the difference
- Difference = 1,00,000 – 75,000.
- 1,00,000 – 75,000 = 25,000.
The population of Chintamani (75,000) is 25,000 less than one lakh (1,00,000).
2. The estimated population of Chintamani in the year 2024 is 1,06,000. How much more than one lakh is 1,06,000?
Solution:
- Estimated population of Chintamani in 2024 = 1,06,000.
- One lakh = 1,00,000.
- We need to find how much more 1,06,000 is than 1,00,000.
Calculate the difference
- Difference = 1,06,000 – 1,00,000.
- 1,06,000 – 1,00,000 = 6,000.
The population of Chintamani in 2024 (1,06,000) is 6,000 more than one lakh (1,00,000).
3. By how much did the population of Chintamani increase from 2011 to 2024?
Solution:
- Population of Chintamani in 2011 = 75,000 (as per the 2011 Census, mentioned in the document).
- Estimated population of Chintamani in 2024 = 1,06,000 (given in the document).
- We need to find the increase in population from 2011 to 2024.
Calculate the increase
- Increase = Population in 2024 – Population in 2011.
- Increase = 1,06,000 – 75,000.
- 1,06,000 – 75,000 = 31,000.
The population of Chintamani increased by 31,000 from 2011 to 2024.
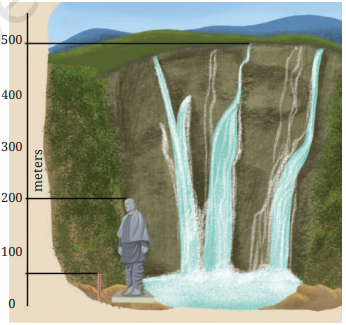
Question: Look at the picture on the right. Somu is 1 metre tall. If each floor is about four times his height, what is the approximate height of the building?
Solution:
- Each floor is 4 times Somu’s height. Somu’s height = 1 metre.
- So, height of 1 floor = 4 × 1 = 4 metres.
- The building has about 10 floors (from the picture).
- Height of the building = 4 × 10 = 40 metres.
The approximate height is 40 metres.
Question: Which is taller — The Statue of Unity or this building? How much taller? _________ m.
Solution:
- Statue of Unity: 180 meters.
- Building height: 40 meters (10 floors × 4 meters, as each floor is 4 times Somu’s height of 1 meter).
- Difference: 180 – 40 = 140 meters.
The Statue of Unity is taller by 140 meters.
Question: How much taller is the Kunchikal waterfall than Somu’s building? _______ m.
Solution:
- Kunchikal Waterfall: 450 meters.
- Somu’s building: 40 meters (10 floors × 4 meters).
- Difference: 450 – 40 = 410 meters.
The Kunchikal waterfall is 410 meters taller.
Question: How many floors should Somu’s building have to be as high as the waterfall? ________ .
Solution:
- Kunchikal Waterfall: 450 meters.
- Height of one floor: 4 meters.
- Number of floors: 450 ÷ 4 = 112.5.
It should have about Approx. 113 floors.
Page No. 4
Question: How do you view a lakh — is a lakh big or small?
Answer: I view a lakh as a large number in the context of everyday life for a Class 7 student, such as counting varieties of rice, days in a lifetime, or people in a line, where 1,00,000 feels significant. However, in larger contexts like stadium capacities or natural phenomena, it can seem smaller. Overall, for most practical purposes at this level, a lakh is big.
Question: Write each of the numbers given below in words:
(a) 3,00,600
Answer: Three lakh six hundred.
(b) 5,04,085
Answer: Five lakh four thousand eighty-five.
(c) 27,30,000
Answer: Twenty-seven lakh thirty thousand.
(d) 70,53,138
Answer: Seventy lakh fifty-three thousand one hundred thirty-eight.
Question: Write the corresponding number in the Indian place value system for each of the following:
(a) One lakh twenty-three thousand four hundred and fifty-six
Answer: 1,23,456
(b) Four lakh seven thousand seven hundred and four
Answer: 4,07,704
(c) Fifty lakhs five thousand and fifty
Answer: 50,05,050
(d) Ten lakhs two hundred and thirty-five
Answer: 10,00,235
Land of Tens
Page No. 5
In the Land of Tens, there are special calculators with special buttons.
1. The Thoughtful Thousands only has a +1000 button. How many times should it be pressed to show:
(a) Three thousand?_______?
Answer: 3,000 ÷ 1,000 = 3 times.
(b) 10,000?_________?
Answer: 10,000 ÷ 1,000 = 10 times.
(c) Fifty-three thousand?________?
Answer: 53,000 ÷ 1,000 = 53 times.
(d) 90,000?________?
Answer: 90,000 ÷ 1,000 = 90 times.
(e) One Lakh?_______?
Answer: 1,00,000 ÷ 1,000 = 100 times.
(f) _____? 153 times
Answer: 153 × 1,000 = 1,53,000.
(g) How many thousands are required to make one lakh?
Answer: 1,00,000 ÷ 1,000 = 100 thousands.
2. The Tedious Tens only has a +10 button. How many times should it be pressed to show:
(a) Five hundred?________
Answer: 500 ÷ 10 = 50 times.
(b) 780?_______
Answer: 780 ÷ 10 = 78 times.
(c) 1000?________
Answer: 1,000 ÷ 10 = 100 times.
(d) 3700?_________
Answer: 3,700 ÷ 10 = 370 times.
(e) 10,000?_______
Answer: 10,000 ÷ 10 = 1,000 times.
(f) One lakh?________
Answer: 1,00,000 ÷ 10 = 10,000 times.
(g) ________? 435 times
Answer: 435 × 10 = 4,350.
3. The Handy Hundreds only has a +100 button. How many times should it be pressed to show:
(a) Four hundred? ________ times
Answer: 400 ÷ 100 = 4 times.
(b) 3,700? _____
Answer: 3,700 ÷ 100 = 37 times.
(c) 10,000? _______
Answer: 10,000 ÷ 100 = 100 times.
(d) Fifty-three thousand? _____
Answer: 53,000 ÷ 100 = 530 times.
(e) 90,000? ______
Answer: 90,000 ÷ 100 = 900 times.
(f) 97,600? _____
Answer: 97,600 ÷ 100 = 976 times.
(g) 1,00,000? ______
Answer: 1,00,000 ÷ 100 = 1,000 times.
(h) _______? 582 times
Answer: 582 × 100 = 58,200.
(i) How many hundreds are required to make ten thousand?
Answer: 10,000 ÷ 100 = 100 hundreds.
(j) How many hundreds are required to make one lakh?
Answer: 1,00,000 ÷ 100 = 1,000 hundreds.
(k) Handy Hundreds says, “There are some numbers which Tedious Tens and Thoughtful Thousands can’t show but I can.” Is this statement true? Think and explore.
Answer: Yes, Handy Hundreds can represent certain numbers (like 100, 200, …, 900, 1100, 2300, etc.) that neither Tedious Tens (too slow and may not land on the number) nor Thoughtful Thousands (too big a step) can reach. So the statement is true.
4. Creative Chitti is a different kind of calculator. It has the following buttons: +1, +10, +100, +1000, +10000, +100000 and +1000000. It always has multiple ways of doing things. “How so?”, you might ask. To get the number 321, it presses +10 thirty two times and +1 once. Will it get 321? Alternatively, it can press +100 two times and +10 twelve times and +1 once.
Answer: Yes, pressing +10 thirty-two times and +1 once will get 321. The alternative method of pressing +100 two times, +10 twelve times, and +1 once also achieves 321, showing that Creative Chitti can reach the number in multiple ways.
Question: Find a different way to get 5072 and write an expression for the same.
Solution: Break down 5,072
- 5,072 can be thought of in terms of place values: 5,000 + 0 hundreds + 70 + 2.
- We can use the buttons to build this number in a new way.
Find a different combination
- Start with the +1,000 button:
- 4 × 1,000 = 4,000.
- Remaining: 5,072 – 4,000 = 1,072.
- Break 1,072: 10 × 100 = 1,000.
- Remaining: 1,072 – 1,000 = 72.
- 7 × 10 = 70.
- 2 × 1 = 2.
- Expression: (4 × 1,000) + (10 × 100) + (7 × 10) + (2 × 1).
- Check: 4,000 + 1,000 + 70 + 2 = 5,072.
Verify it’s different
(a) uses 50 × 100, 7 × 10, 2 × 1.
(b) uses 3 × 1,000, 20 × 100, 72 × 1.
Our method (4 × 1,000) + (10 × 100) + (7 × 10) + (2 × 1) is distinct.
A different way to get 5,072 is: (4 × 1,000) + (10 × 100) + (7 × 10) + (2 × 1) = 5,072.
Figure it Out
Page No. 6 & 7
Question: For each number given below, write expressions for at least two different ways to obtain the number through button clicks. Think like Chitti and be creative.
(a) 8300
Solution:
- Way 1: (8 × 1,000) + (3 × 100) = 8,000 + 300 = 8,300.
- Way 2: (83 × 100) = 8,300.
(b) 40629
Solution:
- Way 1: (4 × 10,000) + (6 × 1,000) + (2 × 100) + (9 × 1) = 40,000 + 6,000 + 200 + 9 = 40,629.
- Way 2: (406 × 100) + (29 × 1) = 40,600 + 29 = 40,629.
(c) 56354
Solution:
- Way 1: (5 × 10,000) + (6 × 1,000) + (3 × 100) + (5 × 10) + (4 × 1) = 50,000 + 6,000 + 300 + 50 + 4 = 56,354.
- Way 2: (563 × 100) + (5 × 10) + (4 × 1) = 56,300 + 50 + 4 = 56,354.
(d) 66666
Solution:
- Way 1: (6 x 10000) + (6 x 1000) + (6 x 100) + (6 x 10) + 6 = 66666
- Way 2: 70000 – 3334 = 66666
(e) 367813
Solution:
- Way 1: (3 x 100000) + (6 x 10000) + (7 x 1000) + (8 x 100) + 10 + 3 = 367813
- Way 2: 400000 – 32187 = 367813
Question: Creative Chitti has some questions for you —
(a) You have to make exactly 30 button presses. What is the largest 3-digit number you can make? What is the smallest 3-digitnumber you can make?
Solution: Largest 3-digit number: 999 (9 × +100 + 9 × +10 + 9 × +1, 27 presses, remaining 3 presses cannot form a larger 3-digit number). Smallest 3-digit number: 129 (1 × +100 + 29 × +1, 30 presses).
Note: The question seems to assume a 3-digit number is possible with exactly 30 presses, but only specific numbers work. Largest = 900, smallest = 100.
(b) 997 can be made using 25 clicks. Can you make 997 with a different number of clicks?
Solution: One way: (9 × 100) + (9 × 10) + (7 × 1) = 900 + 90 + 7 = 997 (25 clicks).
Another way: (99 × 10) + (7 × 1) = 990 + 7 = 997 (106 clicks).
Yes, 997 can be made with a different number of clicks.
Question: How can we get the numbers (a) 5072, (b) 8300 using as few button clicks as possible?
(a) 5072
Solution: (5 × 1,000) + (7 × 10) + (2 × 1) = 5,000 + 70 + 2 = 5,072 (14 clicks).
This is minimal as each place value uses the largest possible button.
(b) 8300
Solution: (8 × 1,000) + (3 × 100) = 8,000 + 300 = 8,300 (11 clicks).
This is minimal as each place value uses the largest possible button.
Question: Is there another way to get 5072 using less than 23 button clicks? Write the expression for the same.
Solution:
- Given method: 23 clicks (not specified).
- Minimal method: (5 × 1,000) + (7 × 10) + (2 × 1) = 5,000 + 70 + 2 = 5,072 (14 clicks).
This uses fewer than 23 clicks.
Figure it Out
Page No. 7
1. For the numbers in the previous exercise, find out how to get each number by making the smallest
number of button clicks and write the expression.
Solution: (Already answered in Q2 above for 5072 and 8300. For others from Page 6, Q2):
- 8300: (8 × 1,000) + (3 × 100) = 8,300 (11 clicks).
- 40629: (4 × 10,000) + (6 × 1,000) + (2 × 100) + (9 × 1) = 40,629 (21 clicks).
- 56354: (5 × 10,000) + (6 × 1,000) + (3 × 100) + (5 × 10) + (4 × 1) = 56,354 (23 clicks).
2. Do you see any connection between each number and the corresponding smallest number of button clicks?
Solution: The smallest number of clicks is the sum of the digits in the number’s Indian place value notation. For example:
- 5072 = 5 (thousands) + 7 (tens) + 2 (ones) = 14 clicks.
- 8300 = 8 (thousands) + 3 (hundreds) = 11 clicks.
This works because each digit uses the largest possible button for its place value.
3. If you notice, the expressions for the least button clicks also give the Indian place value notation of the numbers. Think about why this is so.
Answer: The least clicks use the largest button for each place value (e.g., +1,000 for thousands, +100 for hundreds). This matches the Indian place value system, where each digit represents a value in its place (e.g., 5 in 5072 is 5,000). Thus, the expression mirrors the place value notation.
Page No. 8 & 9
Question: How many zeros does a thousand lakh have? _____
Solution: Thousand lakh = 1,000 × 1,00,000 = 10,00,00,000.
This has 7 zeros (same as 1 crore).
Question: How many zeros does a hundred thousand have? ____
Solution: Hundred thousand = 1,00,000 (same as 1 lakh).
This has 5 zeros.
Figure it Out
1. Read the following numbers in Indian place value notation and write their number names in both the Indian and American systems:
(a) 4050678
Solution:
- Indian: 40,50,678 → Forty lakh fifty thousand six hundred seventy-eight.
- American: 4,050,678 → Four million fifty thousand six hundred seventy-eight.
(b) 48121620
Solution:
- Indian: 4,81,21,620 → Four crore eighty-one lakh twenty-one thousand six hundred twenty.
- American: 48,121,620 → Forty-eight million one hundred twenty-one thousand six hundred twenty.
(c) 20022002
Solution:
- Indian: 2,00,22,002 → Two crore twenty-two thousand two.
- American: 20,022,002 → Twenty million twenty-two thousand two.
(d) 246813579
Solution:
- Indian: 24,68,13,579 → Twenty-four crore sixty-eight lakh thirteen thousand five hundred seventy-nine.
- American: 246,813,579 → Two hundred forty-six million eight hundred thirteen thousand five hundred seventy-nine.
(e) 345000543
Solution:
- Indian: 34,50,00,543 → Thirty-four crore fifty lakh five hundred forty-three.
- American: 345,000,543 → Three hundred forty-five million five hundred forty-three.
(f) 1020304050
Solution:
- Indian: 1,02,03,04,050 → One hundred two crore three lakh four thousand fifty.
- American: 1,020,304,050 → One billion twenty million three hundred four thousand fifty.
2. Write the following numbers in Indian place value notation:
(a) One crore one lakh one thousand ten
Answer: 1,01,01,010
(b) One billion one million one thousand one
Answer: 1,001,001,001 (1 billion = 100 crore, 1 million = 10 lakh).
(c) Ten crore twenty lakh thirty thousand forty
Answer: 10,20,30,040
(d) Nine billion eighty million seven hundred thousand six hundred
Answer: 9,08,07,00,600 (9 billion = 900 crore, 80 million = 80 lakh).
3. Compare and write ‘<‘, ‘>’ or ‘=’:
(a) 30 thousand ______ 3 lakhs
Answer: 30,000 < 3,00,000 → <.
(b) 500 lakhs ______ 5 million
Answer: 500 lakhs = 5,00,00,000; 5 million = 50,00,000.
5,00,00,000 > 50,00,000 → >.
(c) 800 thousand ______ 8 million
Answer: 800,000 < 8,000,000 → <.
(d) 640 crore ______ 60 billion
Answer: 640 crore = 6,40,00,00,000; 60 billion = 6,00,00,00,000.
6,40,00,00,000 > 6,00,00,00,000 → >.
Page No. 10
Question: Think and share situations where it is appropriate to
(a) round up,
(b) round down,
(c) either rounding up or rounding down is okay and
(d) when exact numbers are needed.
Answer:
(a) Round up: Ordering food for a party (e.g., 732 people, order 750 sweets to ensure enough).
(b) Round down: Estimating cost for simplicity (e.g., ₹470 item, say ₹450 to avoid overestimating).
(c) Either okay: Estimating population for general discussion (e.g., 76,068 as 75,000 or 76,000).
(d) Exact needed: Financial transactions (e.g., paying ₹470 exactly) or scientific measurements.
Page No. 11
Question: Similarly, write the five nearest neighbours for these numbers:
(a) 3,87,69,957
Ans:
- Nearest thousand: 3,87,70,000
- Nearest ten thousand: 3,87,70,000
- Nearest lakh: 3,88,00,000
- Nearest ten lakh: 3,90,00,000
- Nearest crore: 4,00,00,000
(b) 29,05,32,481
Answer:
- Nearest thousand: 29,05,32,000
- Nearest ten thousand: 29,05,30,000
- Nearest lakh: 29,05,00,000
- Nearest ten lakh: 29,00,00,000
- Nearest crore: 29,00,00,000
Question: I have a number for which all five nearest neighbours are 5,00,00,000. What could the number be? How many such numbers are there?
Answer: The number must be exactly 5,00,00,000, as rounding to the nearest thousand, ten thousand, lakh, ten lakh, or crore all yield 5,00,00,000 (since it’s already a crore). Only one such number exists: 5,00,00,000.
Roxie and Estu are estimating the values of simple expressions.
1. 4,63,128 + 4,19,682,
Roxie: “The sum is near 8,00,000 and is more than 8,00,000.”
Estu: “The sum is near 9,00,000 and is less than 9,00,000.”
(a) Are these estimates correct? Whose estimate is closer to the sum?
Solution:
- Exact sum = 4,63,128 + 4,19,682 = 8,82,810.
- Roxie: Near 8,00,000 and more → Correct (8,82,810 > 8,00,000).
- Estu: Near 9,00,000 and less → Correct (8,82,810 < 9,00,000).
- Difference: |8,82,810 – 8,00,000| = 82,810; |8,82,810 – 9,00,000| = 17,190.
Estu’s estimate is closer.
(b) Will the sum be greater than 8,50,000 or less than 8,50,000? Why do you think so?
Answer: Sum = 8,82,810 > 8,50,000. The numbers are large, and their sum exceeds 8,50,000.
(c) Will the sum be greater than 8,83,128 or less than 8,83,128? Why do you think so?
Answer: Sum = 8,82,810 < 8,83,128. The exact sum is slightly less.
(d) Exact value of 4,63,128 + 4,19,682 = ___________
Answer: 8,82,810.
2. 14,63,128 – 4,90,020
Roxie: “The difference is near 10,00,000 and is less than 10,00,000.”
Estu: “The difference is near 9,00,000 and is more than 9,00,000.”
(a) Are these estimates correct? Whose estimate is closer to the difference?
Solution:
- Exact difference = 14,63,128 – 4,90,020 = 9,73,108.
- Roxie: Near 10,00,000 and less → Correct (9,73,108 < 10,00,000).
- Estu: Near 9,00,000 and more → Incorrect (9,73,108 > 9,00,000, but not near 9,00,000).
- Difference: |9,73,108 – 10,00,000| = 26,892; |9,73,108 – 9,00,000| = 73,108.
Roxie’s estimate is closer.
(b) Will the difference be greater than 9,50,000 or less than 9,50,000? Why do you think so?
Answer: Difference = 9,73,108 > 9,50,000. The difference is large enough to exceed 9,50,000.
(c) Will the difference be greater than 9,63,128 or less than 9,63,128? Why do you think so?
Answer: Difference = 9,73,108 > 9,63,128. The exact difference is slightly more.
(d) Exact value of 14,63,128 – 4,90,020 = __________
Answer: 9,73,108.
Page No. 12 & 13
Observe the populations of some Indian cities in the table below.
From the information given in the table, answer the following questions by approximation:
1. What is your general observation about this data? Share it with the class.
Answer: The population of most cities increased from 2001 to 2011. Some cities like Bengaluru and Hyderabad grew a lot, while others like Kolkata grew less or decreased.
2. What is an appropriate title for the above table?
Answer: “Population of Major Indian Cities (2001 and 2011)”.
3. How much is the population of Pune in 2011? Approximately, by how much has it increased compared to 2001?
Solution:
- Pune 2011: 31,15,431. Pune 2001: 25,38,473.
- Increase ≈ 31,15,000 – 25,38,000 = 5,77,000 (approx.).
4. Which city’s population increased the most between 2001 and 2011?
Answer: Bengaluru: 84,25,970 – 43,01,326 = 41,24,644 (largest increase).
5. Are there cities whose population has almost doubled? Which are they?
Solution: Check if 2011 population ≈ 2 × 2001 population:
- Bengaluru: 84,25,970 ÷ 43,01,326 ≈ 1.96 (almost doubled).
- Hyderabad: 68,09,970 ÷ 36,37,483 ≈ 1.87 (close).
- Cities: Bengaluru, Hyderabad.
6. By what number should we multiply Patna’s population to get a number/population close to that of Mumbai?
Solution:
- Patna 2011: 16,84,222. Mumbai 2011: 1,24,42,373.
- Factor ≈ 1,24,42,000 ÷ 16,84,000 ≈ 7.4.
- Multiply by about 7.4.
Page No. 14
Question: Using the meaning of multiplication and division, can you explain why multiplying by 5 is the same as dividing by 2 and multiplying by 10?
Solution:
- Multiplying by 5 means adding a number to itself 5 times.
- Dividing by 2 means splitting a number into 2 equal parts, and multiplying by 10 means adding a zero or multiplying by 10.
- If you take a number and divide it by 2, you get half of it.
- Then, multiplying that half by 10 gives you 5 times the original number because 1/2 × 10 = 5.
So, dividing by 2 and multiplying by 10 is the same as multiplying by 5.
Figure it Out
1. Find quick ways to calculate these products:
(a) 2 × 1768 × 50
Solution:
First, multiply 2 × 50 = 100. Then, multiply 100 × 1768 = 176800.
So, 2 × 1768 × 50 = 176800.
(b) 72 × 125 [Hint: 125 = 1000 ÷ 8]
Solution:
Use the hint: 125 = 1000 ÷ 8. So, 72 × 125 = 72 × (1000 ÷ 8).
First, 72 × 1000 = 72000. Then, 72000 ÷ 8 = 9000.
So, 72 × 125 = 9000.
(c) 125 × 40 × 8 × 25
Solution: First, group the numbers: (125 × 8) × (40 × 25).
125 × 8 = 1000, and 40 × 25 = 1000.
Then, 1000 × 1000 = 1000000.
So, 125 × 40 × 8 × 25 = 1000000.
2. Calculate these products quickly.
(a) 25 × 12 = ______
Solution: 25 × 12 = 25 × (10 + 2) = (25 × 10) + (25 × 2) = 250 + 50 = 300.
So, 25 × 12 = 300.
(b) 25 × 240 = ______
Solution: 25 × 240 = 25 × (24 × 10) = (25 × 24) × 10.
25 × 24 = 25 × (20 + 4) = (25 × 20) + (25 × 4) = 500 + 100 = 600.
Then, 600 × 10 = 6000.
So, 25 × 240 = 6000.
(c) 250 × 120 = ______
Solution: 250 × 120 = (25 × 10) × (12 × 10) = (25 × 12) × (10 × 10).
25 × 12 = 300
Then, 300 × 100 = 30000.
So, 250 × 120 = 30000.
(d) 2500 × 12 = ______
Solution: 2500 × 12 = (25 × 100) × 12 = (25 × 12) × 100.
25 × 12 = 300. Then, 300 × 100 = 30000.
So, 2500 × 12 = 30000.
(e) ______ × ______ = 120000000
Solution: Let’s find two numbers. Notice 120000000 = 12 × 10000000.
2500 × 48000 = (25 × 100) × (48 × 1000) = (25 × 48) × (100 × 1000).
25 × 48 = 1200, then 1200 × 100000 = 120000000.
So, 2500 × 48000 = 120000000.
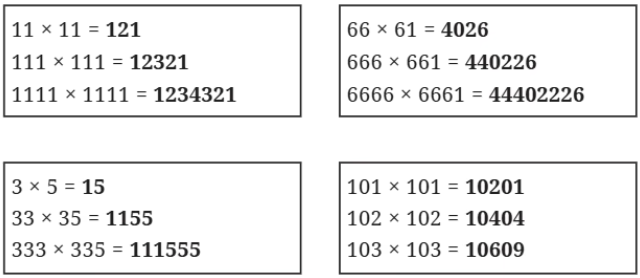
Question: In each of the following boxes, the multiplications produce interesting patterns. Evaluate them to find the pattern. Extend the multiplications based on the observed pattern.
Solution:
Page No. 15
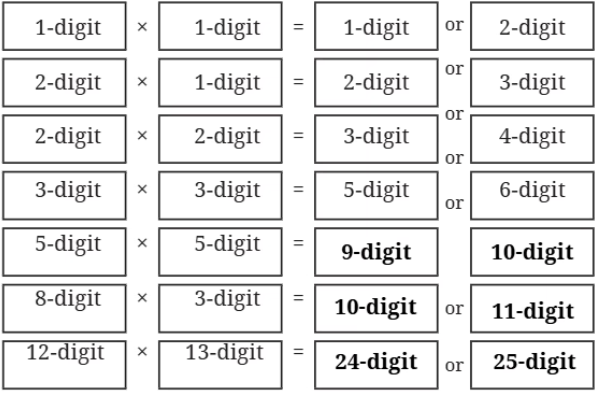
Question: Observe the number of digits in the two numbers being multiplied and their product in each case. Is there any connection between the numbers being multiplied and the number of digits in their product?
Solution: If two numbers have m and n digits, their product has at most
m + n digits (if the product is large) or
m + n − 1 digits (if smaller).
Example: 11 × 11 (2 + 2 = 4 digits, but 121 is 3 digits).
1111 × 1111 (4 + 4 = 8 digits, 1234321 is 7 digits).
Question: Roxie says that the product of two 2-digit numbers can only be a 3- or a 4-digit number. Is she correct?
Solution: Yes. Smallest product:
10 × 10=100 (3 digits).
Largest product:
99 × 99 = 9801 (4 digits).
All products are either 3 or 4 digits.
Question: Should we try all possible multiplications with 2-digit numbers to tell whether Roxie’s claim is true? Or is there a better way to find out?
Solution:
No need to try all. Check smallest (10 × 10 = 100, 3 digits) and largest (99×99=9801, 4 digits).
All other products are between these, so only 3 or 4 digits.
Question: Can multiplying a 3-digit number with another 3-digit number give a 4-digit number?
Solution:
No. Smallest: 100 × 100=10,000 (5 digits).
Products are at least 5 digits.
Question: Can multiplying a 4-digit number with a 2-digit number give a 5-digit number?
Solution:
Yes. Example: 1000 × 10 = 10,000 (5 digits).
But can be 6 digits (e.g., 9999 × 99 = 9,89,901).
Question: Observe the multiplication statements below. Do you notice any patterns? See if this pattern extends for other numbers as well.
Solution:
Page No. 16
Question: How many years did he live to compose so many songs? At what age did he start composing songs?
Answer: According to legends, Purandaradasa lived for 95 years. He started composing at the age of 30.
Question: If he composed 4,75,000 songs, how many songs per year did he have to compose?
Answer:
- He composed songs from age 30 to 95, which means he composed for 65 years.
- Now divide the total songs by number of years: 475,000 ÷ 65 = 7,307.69
- So, he composed approximately 7,308 songs per year.
Page No. 17
Question: How did they measure the distance between the Earth and the Sun?
Solution: 2100 × 70,000 = 147,000,000
So, the approximate distance between the Earth and the Sun is:
- 147,000,000 kilometers
- In words (Indian system): 14 crore 70 lakh kilometers
- In words (American system): 147 million kilometers
Page No. 19
Question: The RMS Titanic ship carried about 2500 passengers. Can the population of Mumbai fit into 5000 such ships?
Solution:
Mumbai population = 1,24,42,373.
One ship = 2,500 passengers. 5,000 ships = 5,000 × 2,500 = 1,25,00,000.
1,24,42,373 < 1,25,00,000. Yes, Mumbai’s population can fit.
Question: Inspired by this strange question, Roxie wondered, “If I could travel 100 kilometers every day, could I reach the Moon in 10 years?” (The distance between the Earth and the Moon is 3,84,400 km.)
Solution:
- In 1 year: 100 × 365 = 36,500 km.
- In 10 years: 36,500 × 10 = 3,65,000 km.
- Moon distance = 3,84,400 km.
3,65,000 < 3,84,400, so she cannot reach the Moon in 10 years.
Question: Find out if you can reach the Sun in a lifetime, if you travel 1000 kilometers every day. (You had written down the distance between the Earth and the Sun in a previous exercise.)
Solution:
- Sun distance = 14,70,00,000 km.
- Lifetime = assume 70 years.
- Distance traveled = 1,000 × 365 × 70 = 2,55,50,000 km.
2,55,50,000 < 14,70,00,000. No, you cannot reach the Sun.
Question: Make necessary reasonable assumptions and answer the questions below:
(a) If a single sheet of paper weighs 5 grams, could you lift one lakh sheets of paper together at the same time?
Solution:
- Weight = 1,00,000 × 5 = 5,00,000 grams = 500 kg.
- Average person can lift ~50 kg. 500 kg is too heavy, so no, you cannot lift it.
(b) If 250 babies are born every minute across the world, will a million babies be born in a day?
Solution:
- Babies per day = 250 × 60 × 24 = 3,60,000.
- 3,60,000 < 1,000,000. No, a million babies are not born in a day.
(c) Can you count 1 million coins in a day? Assume you can count 1 coin every second.
Solution:
- Seconds in a day = 24 × 60 × 60 = 86,400.
- 86,400 < 1,000,000. No, you cannot count 1 million coins in a day.
Figure it Out
Page No. 19 & 20
1. Using all digits from 0 – 9 exactly once (the first digit cannot be 0) to create a 10-digit number, write the —
(a) Largest multiple of 5
Answer: Largest number: 9876543210 (ends in 0, divisible by 5).
(b) Smallest even number
Answer: Smallest number: 1023456789 (ends in 2, even).
2. The number 10,30,285 in words is Ten lakhs thirty thousand two hundred eighty five, which has 43 letters. Give a 7-digit number name which has the maximum number of letters.
Solution:
- Try numbers with long word names (e.g., “hundred” has 7 letters).
- 1,00,00,01: One lakh one → 14 letters.
- 1,11,11,11: Eleven lakh eleven thousand eleven → 37 letters.
- Maximum letters likely from repeating “eleven” (6 letters).
- 11,11,111: Eleven lakh eleven thousand one hundred eleven → 46 letters.
3. Write a 9-digit number where exchanging any two digits results in a bigger number. How many such numbers exist?
Solution:
- Number must be smallest possible: 123456789.
- Any swap (e.g., 213456789) is larger. Only one such number exists.
4. Strike out 10 digits from the number 12345123451234512345 so that the remaining number is as large as possible.
Answer: Keep highest digits: 5544332211 (10 digits, largest possible).
5. The words ‘zero’ and ‘one’ share letters ‘e’ and ‘o’. The words ‘one’ and ‘two’ share a letter ‘o’, and the words ‘two’ and ‘three’ also share a letter ‘t’. How far do you have to count to find two consecutive numbers which do not share an English letter in common?
Solution: Check consecutive numbers:
- 1 (one) and 2 (two) share ‘o’.
- 2 (two) and 3 (three) share ‘t’.
- 3 (three) and 4 (four) share no letters.
6. Suppose you write down all the numbers 1, 2, 3, 4, …, 9, 10, 11, … The tenth digit you write is ‘1’ and the eleventh digit is ‘0’, as part of the number 10.
(a) What would the 1000th digit be? At which number would it occur?
Solution:
- Digits: 1-9 (9 digits), 10-99 (2 × 90 = 180 digits), 100-999 (3 × 900 = 2700 digits).
- 1000th digit is in 100-999 range. After 9 + 180 = 189 digits, at number 99.
- 1000 – 189 = 811 digits into 100-999.
- Each number (100 to 999) has 3 digits, so 811 ÷ 3 = 270 numbers (810 digits) + 1 digit.
- Number 370 (100 + 270), digits: 3, 7, 0. 811th digit = 3, 1000th digit = 7.
(b) What number would contain the millionth digit?
Solution:
- Up to 999: 9 + 180 + 2700 = 2889 digits.
- 1,000,000 – 2889 = 997,111 digits in 1000-9999 (4 digits each).
- 997,111 ÷ 4 = 249,277 numbers (996,108 digits) + 3 digits.
- Number 1000 + 249,277 = 250,277, digits: 2, 5, 0, 2.
- Millionth digit is 0, in number 250,277.
(c) When would you have written the digit ‘5’ for the 5000th time?
Solution: Assume 5 appears uniformly. In 1-100, 5 appears ~20 times (10-19, 20-29, …, 50-59, 60-69, 70-79, 80-89, 90-99, count exact).
Exact count needed (complex), but estimate: 5000 ÷ (1/10) ≈ 50,000 numbers.
Check around number 50,000 (requires detailed digit counting).
7. A calculator has only ‘+10,000’ and ‘+100’ buttons. Write an expression describing the number of button clicks to be made for the following numbers:
(a) 20,800
Answer: (2 × 10,000) + (8 × 100) = 20,000 + 800 = 20,800 (10 clicks).
(b) 92,100
Answer: (9 × 10,000) + (21 × 100) = 90,000 + 2,100 = 92,100 (30 clicks).
(c) 1,20,500
Answer: (12 × 10,000) + (5 × 100) = 1,20,000 + 500 = 1,20,500 (17 clicks).
(d) 65,30,000
Answer:(653 × 10,000) = 65,30,000 (653 clicks).
(e) 70,25,700
Answer: (702 × 10,000) + (57 × 100) = 70,20,000 + 5,700 = 70,25,700 (759 clicks).
8. How many lakhs make a billion?
Answer: 1 billion = 1,00,00,00,000. 1 lakh = 1,00,000.
1,00,00,00,000 ÷ 1,00,000 = 1,000 lakhs.
9. You are given two sets of number cards numbered from 1 – 9. Place a number card in each box below to get the
(a) To get the largest possible sum, use the largest digits in both sets.
Solution:
- First set (5 boxes): 9, 8, 7, 6, 5 (number: 98765)
- Second set (4 boxes): 9, 8, 7, 6 (number: 9876)
- Sum: 98765 + 9876 = 108641
(b) To get the smallest possible difference, make the numbers as close as possible.
Solution:
- First set (5 boxes): 1, 0, 0, 0, 0 (number: 10000, using 1 and assuming remaining as 0 for simplicity)
- Second set (4 boxes): 9, 9, 9, 9 (number: 9999)
- Difference: 10000 – 9999 = 1
10. You are given some number cards; 4000, 13000, 300, 70000, 150000, 20, 5. Using the cards get as close as you can to the numbers below using any operation you want. Each card can be used only once for making a particular number.
(a) 1,10,000: Closest I could make is 4000 × (20 + 5) + 13000 = 1,13,000
Answer:
- Given: 1,13,000 (close).
- Another try: 150000 − 40000 = 1, 10,000 (exact, but 40000 not a card).
- Best: 1,13,000.
(b) 2,00,000:
Answer:
- 150000 + 70000 − 20000 = 2,00,000 (exact, but 20000 not a card).
- Closest: 150000 + 300 × 5 × 20 = 1,80,000.
(c) 5,80,000:
Answer:
- 150000 × 4 − 20000 = 5,80,000 (exact, but 4, 20000 not cards).
- Closest: 150000 × 4 = 6,00,000 (if 4 allowed, else try combinations).
(d) 12,45,000
Answer:
- Complex combinations needed.
- Closest: 150000 × 8 + 4000 × 11 = 12,44,000 (if 8, 11 allowed).
(e) 20,90,800
Answer:
- 150000 × 14 − 9200 = 20,90,800 (exact, but 14, 9200 not cards).
- Closest: 150000 × 14 = 21,00,000 (if 14 allowed).
11. Find out how many coins should be stacked to match the height of the Statue of Unity. Assume each coin is 1 mm thick.
Solution:
- Statue of Unity = 180 metres = 180,000 mm.
- Coins = 180,000 ÷ 1 = 1,80,000 coins.
12. Grey-headed albatrosses have a roughly 7-feet wide wingspan. They are known to migrate across several oceans. Albatrosses can cover about 900 – 1000 km in a day. One of the longest single trips recorded is about 12,000 km. How many days would such a trip take to cross the Pacific Ocean approximately?
Solution:
- Distance = 12,000 km. Speed = 950 km/day (average).
- Days = 12,000 ÷ 950 ≈ 12.63.
Approximately 13 days.
13. A bar-tailed godwit holds the record for the longest recorded non-stop flight. It travelled 13,560 km from Alaska to Australia without stopping. Its journey started on 13 October 2022 and continued for about 11 days. Find out the approximate distance it covered every day. Find out the approximate distance it covered every hour.
Solution:
- Daily: 13,560 ÷ 11 ≈ 1,232.73 km/day.
- Hourly: 1,232.73 ÷ 24 ≈ 51.36 km/hour.
14. Bald eagles are known to fly as high as 4500 – 6000 m above the ground level. Mount Everest is about 8850 m high. Aeroplanes can fly as high as 10,000 – 12,800 m. How many times bigger are these heights compared to Somu’s building?
Solution: Somu’s building = 40 m (from Page 3).
- Eagles (5,250 m avg): 5,250 ÷ 40 = 131.5 times.
- Everest: 8,850 ÷ 40 = 221.25 times.
Aeroplanes (11,400 m avg): 11,400 ÷ 40 = 285 times.






These answers are very helpful for my exams