Page no. 25.16 Formative Assessment_VSA
Q1.
Answer :
What is the meaning of trial?
The word trial means a test of performance, qualities, or suitability.
Definition:
Any particular performance of a random experiment is called a trial. That is, when we perform an experiment it is called a trial of the experiment.
By experiment or trial, we mean a random experiment unless otherwise specified. Where you are required to differentiate between a trial and an experiment, consider the experiment to be a larger entity formed by the combination of a number of trials.
To illustrate the definition, let us take examples:
1. In the experiment of tossing 4 coins, we may consider tossing each coin as a trial and therefore say that there are 4 trials in the experiment.
2. In the experiment of rolling a dice 5 times, we may consider each rolls as a trial and therefore say that there are 5 trials in the experiment.
Note that rolling a dice 5 times is same as rolling 5 dices each one time. Similarly, tossing 4 coins is same as tossing one coin 4 times.
Q2.
Answer :
What are the meanings of elementary event?
The word elementary means simple, non decomposable into elements or other primary constituents and the word event means something that result.
Definition:
An elementary event is any single outcome of a trial. Elementary events are also called simple events.
To illustrate the definition, let us take examples:
1. In the experiment of tossing a coin, the possible outcomes H and T. Any one outcome like H is called an elementary event.
2. In the experiment of rolling a dice, the possible outcomes are 1, 2, 3, 4, 5 and 6. Any one outcome like 4 is called an elementary event.
Note that H stands for getting a head and T stands for getting a tail in the experiment of tossing a coin.
Q3.
Answer :
What are the meanings of event?
The word event means something that result.
Definition:
An event is a collection of outcomes of a trial of a random experiment.
To illustrate the definition, let us take examples:
1. When two coins are tossed simultaneously, the possible outcomes are HH, HT, TH and TT. Any one outcome like HH is called an event (elementary event). The collections like {HH, HT}, {HH, HT, TT} etc are all events (compound event).
2. In the experiment of rolling a dice, the possible outcomes are 1, 2, 3, 4, 5 and 6. Any one outcome like 4 is called an event (elementary event). The collections like {1, 2}, {1, 2, 3}, {2, 5, 6}, {2, 3, 4, 5} etc are all events (compound events).
Note that H stands for getting a head and T stands for getting a tail in the experiment of tossing a coin.
Q4.
Answer :
The probability of an event denotes the relative frequency of occurrence of an experiment’s outcome, when repeating the experiment.
Definition:
The empirical or experimental definition of probability is that if n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials, then the probability of happening of event A is denoted byand is given by
To illustrate the definition, let us take examples:
1. When two coins are tossed simultaneously, the possible outcomes are HH, HT, TH and TT. The total number of trials is 4. Let A be the event of occurring exactly two heads. The number of times A happens is 1. So, the probability of the event A is
2. In the experiment of rolling a dice, the possible outcomes are 1, 2, 3, 4, 5 and 6. Let A be the event of occurring a number greater than 3. The total number of trials is 6. The number of times A happens is 3. So, the probability of the eventA is
Note that H stands for getting a head and T stands for getting a tail in the experiment of tossing a coin.
Q5.
Answer :
The number of white balls is 4. Let the number of red balls is x. Then the total number of trials is.
Let A be the event of drawing a white ball.
The number of times A happens is 4.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have.
But, it is given that. So, we have
Hence the number of red balls is.
Q6.
Answer :
The total number of trials is 100. Let the number of times an even number is obtained is x.
Let A be the event of getting an even number.
The number of times A happens is x.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have.
But, it is given that. So, we have
Hence an even number is obtained 40 times. Consequently, an odd number is obtainedtimes.
Q7.
Answer :
The total number of trials is 200.
Let A be the event of getting at most two heads.
The number of times A happens is.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have.
Q8.
Answer :
The total number of trials is 200.
Let A be the event of getting atleast two heads.
The number of times A happens is.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
Page no. 25.16 Formative Assessment_MCQ
Q1.
Answer :
We have to find the probability of an impossible event.
Note that the number of occurrence of an impossible event is 0. This is the reason that’s why it is called impossible event.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Note that n is a positive integer, it can’t be zero. So, whatever may be the value of n, the probability of an impossible event is.
Hence the correct option is (b).
Q2.
Answer :
We have to find the probability of a certain event.
Note that the number of occurrence of an impossible event is same as the total number of trials. When we repeat the experiment, every times it occurs. This is the reason that’s why it is called certain event.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Note that n is a positive integer, it can’t be zero. So, the probability of an impossible event is.
Hence the correct option is (b).
Q3.
Answer :
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Note that m is always less than or equal to n and n is a positive integers, it can’t be zero. But, m is a non negative integer. So, the maximum value of probability of an event is, which is the probability of a certain event and the minimum value of it is 0, which is the probability of an impossible event. For any other events the value is in between 0 and 1.
Hence the correct option is (c).
Q4.
Answer :
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Note that m is always less than or equal to n and n is a positive integers, it can’t be zero. But, m is a non negative integer. So, the maximum value of probability of an event is, which is the probability of a certain event and the minimum value of it is 0, which is the probability of an impossible event. For any other events the value is in between 0 and 1.
All the options except (c) satisfy the above criteria’s.
Hence the correct option is (c).
Q5.
Answer :
The random experiment is tossing two coins simultaneously.
All the possible outcomes are HH, HT, TH, and TT.
Let A be the event of getting at most one head.
The number of times A happens is 3.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
So, the correct choice is (b).
Page no. 25.17 Formative Assessment_MCQ
Q6.
Answer :
The total number of trials is 1000. Let x be the number of times a tail occurs.
Let A be the event of getting a tail.
The number of times A happens is x.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have.
But, it is given that. So, we have
Hence a tail is obtained 375 times.
Consequently, a head is obtainedtimes.
So, the correct choice is (c).
Q7.
Answer :
The total number of trials is 600.
Let A be the event of getting a prime number (2, 3 and5).
The number of times A happens is.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
So, the correct choice is (a).
Q8.
Answer :
The total number of trials is 6.
Let A be the event that the attendance of a class is more than 75%.
The number of times A happens is 3 (for classes’ VIII, VII and VI).
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
So, the correct choice is (d).
Q9.
Answer :
The total number of trials is 50.
Let A be the event that the number on the picked coin is not a prime.
The prime’s lies in between 51 and 100 are 53, 59, 61, 67, 71, 73, 79, 83, 89 and 97. They are 10 in numbers. Therefore the numbers lies between 51 and 100 and which are not primes are in numbers.
So, the number of times A happens is 40.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
So, the correct choice is (d).
Q10.
Answer :
The total number of trials is 10.
Let A be the event that Ronaldo makes a goal in a penalty kick.
The number of times A happens is 4.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
So, the correct choice is (d).
Page no. 25.13 Ex. 25.1
Q1.
Answer :
The coin is tossed 1000 times. So, the total number of trials is 1000.
Let A be the event of getting a head and B be the event of getting a tail.
The number of times A happens is 455 and the number of times B happens is 545.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
Q2.
Answer :
The total number of trials is 500.
Let A be the event of getting two heads, B be the event of getting one tail and C be the event of getting no head.
The number of times A happens is 95, the number of times B happens is 290 and the number of times C happens is 115.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Therefore, we have
Q3.
Answer :
The total number of trials is 100.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A be the event of getting two heads.
The number of times A happens is 36.
Therefore, we have
(ii) Let B be the event of getting three heads
The number of times B happens is 12.
Therefore, we have
(iii) Let C be the event of getting at least one head.
The number of times C happens is.
Therefore, we have
(iv) Let D be the event of getting more heads than tails.
The number of times D happens is.
Therefore, we have
(v) Let E be the event of getting more tails than heads.
The number of times E happens is.
Therefore, we have
Q4.
Answer :
The total number of trials is 1500.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A be the event of having no girl.
The number of times A happens is 211.
Therefore, we have
(ii) Let B be the event of having one girl.
The number of times B happens is 814.
Therefore, we have
(iii) Let C be the event of having two girls.
The number of times C happens is 475.
Therefore, we have
(iv) Let D be the event of having at most one girl.
The number of times D happens is.
Therefore, we have
(v) Let E be the event of having more girls than boys.
The number of times E happens is 475.
Therefore, we have
Q5.
Answer :
The total number of trials is 30.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A be the event of hitting boundary.
The number of times A happens is 6.
Therefore, we have
(ii) Let B be the event of does not hitting boundary.
The number of times B happens is.
Therefore, we have
Q6.
Answer :
The total number of trials is 5.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A be the event of getting more than 70% marks.
The number of times A happens is 3.
Therefore, we have
(ii) Let B be the event of getting less than 70% marks.
The number of times B happens is 2.
Therefore, we have
(iii) Let C be the event of getting a distinction.
The number of times C happens is 1.
Therefore, we have
Page no. 25.14 Ex. 25.1
Q7.
Answer :
The total number of trials is 200.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A be the event of liking mathematics.
The number of times A happens is 135.
Therefore, we have
(ii) Let B be the event of disliking mathematics.
The number of times B happens is 65.
Therefore, we have
Q8.
Answer :
The total number of trials is 30.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A1 be the event that the blood group of a chosen student is A.
The number of times A1 happens is 9.
Therefore, we have
(iii) Let A2 be the event that the blood group of a chosen student is B.
The number of times A2 happens is 6.
Therefore, we have
(iii) Let A3 be the event that the blood group of a chosen student is AB.
The number of times A3 happens is 3.
Therefore, we have
(iv) Let A4 be the event that the blood group of a chosen student is O.
The number of times A4 happens is 12.
Therefore, we have
Q9.
Answer :
The total number of trials is 11.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Let A1 be the event that the actual weight of a chosen bag contain more than 5 Kg of flour.
The number of times A1 happens is 7.
Therefore, we have
Q10.
Answer :
The total number of trials is 40.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Let A1 be the event that the birth month of a chosen student is august.
The number of times A1 happens is 5.
Therefore, we have
Q11.
Answer :
The total number of trials is 30.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
Let A1 be the event that the concentration of sulphur dioxide in a day is 0.12-0.16 parts per million.
The number of times A1 happens is 2.
Therefore, we have
Q12.
Answer :
The total number of trials is 2400.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A1 be the event that a chosen family earns Rs 10000-13000 per month and owns exactly 2 vehicles.
The number of times A1 happens is 29.
Therefore, we have
(ii) Let A2 be the event that a chosen family earns Rs 16000 or more per month and owns exactly 1 vehicle.
The number of times A2 happens is 579.
Therefore, we have
(iii) Let A3 be the event that a chosen family earns less than Rs 7000 per month and does not owns any vehicles.
The number of times A3 happens is 10.
Therefore, we have
(iv) Let A4 be the event that a chosen family earns Rs 13000-16000 per month and owns more than 2 vehicles.
The number of times A4 happens is 25.
Therefore, we have
(v) Let A5 be the event that a chosen family owns not more than 1 vehicle (may be 0 or 1). In this case the number of vehicles is independent of the income of the family.
The number of times A5 happens is
.
Therefore, we have
(vi) Let A6 be the event that a chosen family owns atleast 1 vehicle (may be 1 or 2 or above 2). In this case the number of vehicles is independent of the income of the family.
The number of times A6 happens is
.
Therefore, we have
Page no. 25.15 Ex. 25.1
Q13.
Answer :
The total number of trials is 400.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A1 be the event that the lifetime of a chosen bulb is less than 400 hours.
The number of times A1 happens is 14.
Therefore, we have
(ii) Let A2 be the event that the lifetime of a chosen bulb is in between 300 to 800 hours.
The number of times A2 happens is.
Therefore, we have
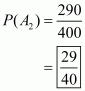
(iii) Let A3 be the event that the lifetime of a chosen bulb is atleast 700 hours.
The number of times A3 happens is.
Therefore, we have
Q14.
Answer :
The total number of trials is 30.
Remember the empirical or experimental or observed frequency approach to probability.
If n be the total number of trials of an experiment and A is an event associated to it such that A happens in m-trials. Then the empirical probability of happening of event A is denoted byand is given by
(i) Let A1 be the event that the wages of a worker is less than Rs 150.
The number of times A1 happens is.
Therefore, we have.
(ii) Let A2 be the event that the wages of a worker is atleast Rs 210.
The number of times A2 happens is.
Therefore, we have.
(iii) Let A3 be the event that the wages of a worker is more than or equal to Rs 150 but less than Rs 210.
The number of times A3 happens is.
Therefore, we have
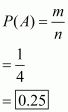


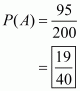
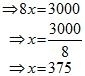
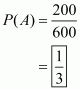
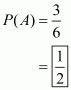
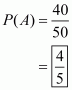
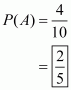
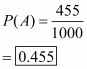
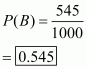
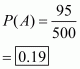
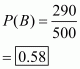
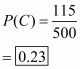
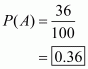
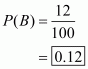
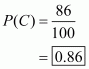
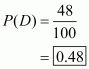
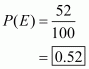
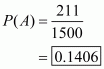

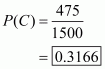
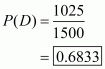

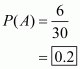
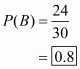
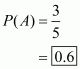
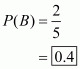
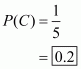
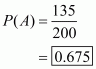
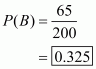
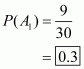
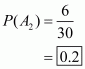

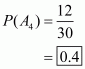
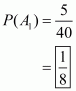
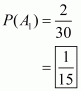
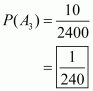
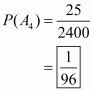
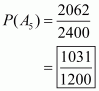
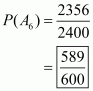
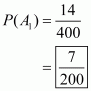

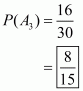

Leave a Reply