A number which divides a given number are 1,2,3 and 6; exact divisors or factors of 15 are 1, exactly is called an exact divisor or factor of that 3, 5 and 15. number.
For example exact divisors or factors of 6 are 1, 2, 3 and 6; exact divisors or factors of 15 are 1, 3, 5 and 15.
Factors and Multiples
A factor of a number is an exact divisor of that number. In turn, a number is a multiple of each of its factors. Some interesting facts about factors and multiples are as follows:
- 1 is a factor of every number.
- Every number is a factor of itself.
- Every factor of a number is an exact divisor of that number.
- Every factor of a number is less than or equal to that number.
- The factors of a given number are finite in number.
- Every multiple of a number is greater than or equal to that number.
- The multiples of a given number are infinite in number.
- Every number is a multiple of itself.
Perfect number: If the sum of all the factors of a number is equal to twice the number, then that number is called a perfect number.
For example: 28 is a perfect number because all the factors of 28 are 1,2,4,7,14 and 28 whose sum = 1 + 2 + 4 + 7 + 14 + 28 = 56 = 2 × 28, whereas 10 is not a perfect number because all the factors of 10 are 1, 2, 5 and 10 whose sum = 1 + 2 + 5 + 10 = 18 ≠ 2 × 10.
Prime and Composite Numbers
Prime numbers: The numbers having exactly two factors 1 and the number itself are called prime numbers.
For example, 2, 3, 5, 7, 11, etc. are prime numbers.
Composite numbers: The numbers having more than two factors are called composite numbers.
For example 4, 6, 8, 9, 10. etc. are composite numbers.
Note: The number 1 is neither prime nor composite.
Even number: A number which is a multiple of 2 is called an even number.
For example: 2, 4, 6, 8, 10,….
Odd number: A number which is not a multiple of 2 is called an odd number.
For example: 1, 3, 5, 7, 9,…
A number with 0, 2, 4, 6, 8 at the unit’s place is an even number.
So, 250, 2732, 29354, 34596 are even numbers.
Obviously, the numbers 257, 3249, 7321 are odd numbers.
2 is the smallest prime number which is even.
Every prime number except 2 is odd.
Tests for Divisibility of Numbers
A number is divisible by 10 if it has 0 in its units place.
A number is divisible by 5 if it has either 0 or 5 in its units place.
A number is divisible by 2 if it has any of the digits 0, 2, 4, 6 or 8 in its units place.
A number is divisible by 3 if the sum of its digits is a multiple of 3.
A number is divisible by 6 if it is divisible by 2 and 3 both.
A number with 3 or more digits is divisible by 4 if the number formed by its last two digits (i.e., units and tens) is divisible by 4.
A number with 4 or more digits is divisible by 8 if the number formed by its last three digits is divisible by 8.
A number is divisible by 9 if the sum of all the digits of the number is divisible by 9.
A number is divisible by 11 if the difference between the sum of the digits at odd places (from the right) and the sum of the digits at even places (from the right) of the number is either 0 or divisible by 11.
Common Factors and Common Multiples
The factors which are the factors of each of the given numbers are called their common factors.
For example:
Factors of 20 are 1, 2, 4, 5, 10 and 20.
Factors of 48 are 1, 2, 3, 4, 6, 8, 12, 16, 24 and 48.
Clearly, the factors common to the factors of 20 and 48 are 1, 2, 4. These are called common factors of 20 and 48.
Note: Common factors are finite in number.
Co-prime numbers: Two numbers are said to be co-prime if they have 1 as the only common factor.
For example, 8 and 25 are co-prime numbers whereas 4 and 10 are not co-prime numbers.
The multiples which are the multiples of each of the given numbers are called their common multiples.
For example:
Multiples of 2 are 2, 4, 6, 8, 10, 12,
Multiples of 3 are 3, 6, 9, 12, 15, 18,
Clearly, the multiples common to the multiples of 2 and 3 are 6, 12, These are called common multiples of 2 and 3.
Note: Common multiples are infinite in number.
Some More Divisibility Rules
If a number is divisible by another number, then it is also divisible by each of the factors of that number.
For example, 40 is divisible by 20.
Factors of 20 are 1, 2, 4, 5, 10 and 20.
Clearly, 40 is divisible by each of these factors
If a number is divisible by two co-prime numbers, then it is also divisible by their product.
For example, 40 is divisible by 4 and 5. 4 and 5 are co-prime.
Their product is 4 × 5 = 20.
Clearly, 40 is divisible by 20.
If two given numbers are divisible by a number, then their sum is also divisible by that number.
For example, The numbers 10 and 25 are divisible by a number 5.
Their sum is 10 + 25 = 35.
Clearly, 35 is divisible by 5.
If two given numbers are divisible by a number, then their difference is also divisible by that number.
For example, The numbers 10 and 25 are divisible by a number 5.
Their difference is 25 – 10 = 15.
Clearly, 15 is divisible by 5.
Prime Factorisation
Factorisation: A number when expressed as a product of its factors is said to be factorised.
For example: 12 = 3 × 4. We say that 12 has been factorised. This is one of the several factorisations of 12.
The others are:
12 = 2 × 6
12 = 3 × 4
12 = 1 × 12.
Prime Factorisation: It is the ultimate factorisation of a given number. Moreover, it is unique (exactly one).
For example: While factorising 12, we ultimately reach the unique factorisation 2 × 2 × 3. In this factorisation, the only factors 2 and 3 are prime numbers. Such a factorisation of a number is called prime factorisation of that number. Thus, 2 × 2 × 3 is the only prime factorisation of 12.
Prime Factorisation Property
or
The Fundamental Theorem of Arithmetic
Every number greater than 1 has exactly one prime factorisation.
or
The prime factorisation of a number (>1) is unique.
0r
There exists only one prime factorisation of a number.
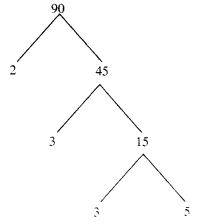
Factor tree: When we go on factorizing a number till we reach its ultimate factorisation and write the process as follows, we get the shape of a tree called Factor tree.
For example: Let us factorize 90. Let us see below:
Thus. 90 = 2 × 3 × 3 × 5.
Highest Common Factor (HCF)
The Highest Common Factor (HCF) of two or more given numbers is the highest (or greatest) of their common factors. It is also known as the Greatest Common Divisor (GCD).
For example: Consider two numbers 12 and 20. Factors of 12 are 1, 2, 3, 4, 6 and 12. Factors of 20 are 1, 2, 4, 5, 10 and 20. The common factors of 12 and 20 are 1, 2 and 4. The highest of these is 4. So, 4 is the HGF of 12 and 20.
Lowest Common Multiple (LCM)
The Lowest Common Multiple (LCM) of two or more given numbers is the lowest (or smallest or least) of their common multiples.
For example: Consider two numbers 12 and 20.
Multiples of 12 are 12, 24, 36, 48, 60, 72, 84, 96, 108, 120, …
Multiples of 20 are 20, 40, 60, 80, 100, 120, ……..
The common multiples of 12 and 20 are 60, 120,…
The lowest of these is 60.
So, 60 is the LCM of 12 and 20.
Some Problems on HCF and LCM
In our everyday life, we are confronted with many situations in which we find it desirable to use the concepts of HCF and LCM.

Leave a Reply