Solutions For All Chapters – Science Curiosity Class 7
Measurement of Time and Motion
1. Calculate the speed of a car that travels 150 metres in 10 seconds. Express your answer in km/h.
Solution:
Distance = 150 m
Time taken = 10 s
Speed = \( \frac{Distance\ covered} {Time\ taken}\)
= \( \frac{150m}{10 s}\)
Speed in km/hr = 15 × \( \frac{18}{5}\)
= 54 km/h
2. A runner completes 400 metres in 50 seconds. Another runner completes the same distance in 45 seconds. Who has a greater speed and by how much?
Solution:
Runner 1:
Distance = 400 m,
Time = 50 s
Speed = \( \frac{Distance\ covered} {Time\ taken}\)
= \( \frac{400m}{50s}\)
= 8 m/s
Runner 2:
Distance = 400 m,
Time = 50 s
Speed = \( \frac{Distance\ covered} {Time\ taken}\)
= \( \frac{400m}{450s}\)
= 8.89 m/s
Difference = 8.89 – 8 = 0.89 m/s
Hence, speed of runner 2 is greater by approximately 0.89 m/s.
3. A train travels at a speed of 25 m/s and covers a distance of 360 km. How much time does it take?
Solution:
Speed = 25 m/s
Distance = 360 km = 3,60,000 m
Time taken = \( \frac{Distance}{Speed}\)
= \( \frac{3,60,0000 m}{25 m/s}\)
= 14,400s
⇒ 240 mins
⇒ 4 hours
4. A train travels 180 km in 3 h. Find its speed in:
(i) km/h
(ii) m/s
(iii) What distance will it travel in 4 h if it maintains the same speed throughout the journey?
Solution:
Distance = 180 km, time = 3h
(i) Speed = \( \frac{Distance}{Time}\)
= \( \frac{180km}{3h}\)
= 60 Km/h
(ii) Speed in m/s= 60 x \( \frac{5}{18}\)
= 16.677 m/s
(iii) Time = 4h, Speed = 60 km/h
Distance = Speed × Time
= 60 × 4 = 240 km
5. The fastest galloping horse can reach the speed of approximately 18 m/s. How does this compare to the speed of a train moving at 72 km/h?
Solution:
Speed of horse = 18 m/s
Speed of train = 72 km/h = 72 × \( \frac{5}{18}\) = 20 m/s
The train is faster by 2 rn/s than the fastest galloping horse.
6. Distinguish between uniform and non-uniform motion using the example of a car moving on a straight highway with no traffic and a car moving in city traffic.
Answer:
| Type of Motion | Example | Description |
|---|---|---|
| Uniform Motion | A car moving on a straight highway with no traffic | The car moves at a constant speed, covering equal distances in equal time intervals. |
| Non-uniform Motion | A car moving in city traffic | The car changes its speed frequently due to signals, turns, and congestion. It covers unequal distances in equal time intervals. |
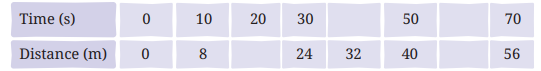
7. Data for an object covering distances in different intervals of time are given in the following table. If the object is in uniform motion, fill in the gaps in the table.
Solution:
In uniform motion, object covers equal distances in equal intervals of time. Hence, the speed of an object remains constant throughout the motion.
| Time (s) | 0 | 10 | 20 | 30 | 40 | 50 | 60 | 70 |
| Distance (m) | 0 | 8 | 16 | 24 | 32 | 40 | 48 | 56 |
The object covers 8 m in every 10 seconds. Hence, the speed of an object remains constant at 0.8 m/s throughout the motion.
8. A car covers 60 km in the first hour, 70 km in the second hour, and 50 km in the third hour. Is the motion uniform? Justify your answer. Find the average speed of the car.
Solution:
Find the average speed of the car.
The car covers different distances in each hour. Hence, the motion of the car is nonuniform.
Total distance = 60 km + 70 km + 50 km = 180 km
Total time = 3 hours
Average speed = \( \frac{Total \ distance \ travelled}{Total \ time \ taken}\)
= \( \frac{180km}{3h}\)
= 60 km/h.
Hence, the average speed of the car is 60 km/h.
9. Which type of motion is more common in daily life-uniform or non-uniform? Provide three examples from your experience to support your answer.
Answer
In our daily life, most motions are nonuniform because object do not move at the same speed all the time. Their speed changes due to factors like traffic, rough or uneven roads and other obstacles.
Examples:
- Travelling in a bus on an uneven road
- Playing cricket
- Walking through a crowded market
10. Data for the motion of an object are given in the following table. State whether the speed of the object is uniform or non-uniform. Find the average speed.
Answer:
| Time interval (s) | Distance (m) |
| 0-10 | 6 – 0 = 6 |
| 10-20 | 10 – 6 = 4 |
| 20-30 | 16 – 10 = 6 |
| 30-40 | 21 – 16 = 5 |
| 40-50 | 29 – 21 = 8 |
| 50-60 | 35 – 29 = 6 |
| 60-70 | 42 – 35 = 7 |
| 70-80 | 45 – 42 = 3 |
| 80-90 | 55 – 45 = 10 |
| 90-100 | 60 – 55 = 5 |
The object exhibits non-uniform motion, because it covers unequal distances in equal time intervals.
Total distance travelled = 60 m
Total time taken = 100 s
Average speed = \( \frac{Total \ distance}{Total \ time \ taken}\)
= \( \frac{60}{100}\)
= 0.6 m/s
11. A vehicle moves along a straight line and covers a distance of 2 km. In the first 500 m, it moves with a speed of 10 m/s and in the next 500 m, it moves with a speed of 5 m/s. With what speed should it move the remaining distance so that the journey is complete in 200 s? What is the average speed of the vehicle for the entire journey?
Solution:
Given
Total distance = 2 km = 2000 m,
Total time = 200
Step 1: Time taken to cover the first 500 m
Time = \( \frac{Distance}{Speed}\)
= \( \frac{500}{10}\)
= 50 s
Step 2: Time taken to cover the next 500 m
Time = \( \frac{500}{10}\)
= 100 s
Step 3: Remaining distance = 2000 – 1000 = 1000 m
Remaining time = 200 – 150 = 50 s
Step 4: Speed required to cover the remaining 1000 m
Speed = \( \frac{1000}{50}\)
= 20 m/s
Step 5:
Average speed = \( \frac{Total \ distance}{Total \ time \ taken}\)
= \( \frac{2000}{200}\)
= 10 m/s


Leave a Reply